Page 108 - Special Issue Modern Trends in Scientific Research and Development, Case of Asia

International Journal of Trend in Scientific Research and Development (IJTSRD) @ www. ijtsrd. com eISSN: 2456-6470

Therefore, the materials in this section can be fully used by Here, this postulate was treated with suspicion, because
students studying the basics of geometry. people experienced the correctness of other Euclid's
postulates on a daily basis in practice.
The second part of mathematics of the book "The Book of
Knowledge" is devoted to arithmetic and consists of seven
sections. The first section describes the types and general
properties of numbers. Here are given the divisions of
numbers into even and odd numbers and their properties.
The second section deals with even numbers. It describes
about even-even numbers, about even-odd and about the
properties of these numbers. The third section talks about
odd numbers and the fact that they come in three forms, as
well as their properties. They are composed of prime,
compound and reciprocal prime numbers. The fourth section
talks about "imperfect" and "perfect" numbers. It describes

the characteristics of division and the properties of numbers
Picture 1 Euclid`s V postulate
into three types based on the equality or inequality of the

sum of divisions of these numbers. The fifth section talks Avicenna proved the fifth postulate by accepting without
about "excess" and "insufficient" relationships and their evidence the statement that "Two lines located on the same
properties. The sixth section is devoted to "complex"
plane and not intersecting with each other are located from
relationships and statements over numerical examples. The each other at the same distance." But this statement is
seventh section describes the types and properties of
equivalent to the fifth postulate.
proportions.

The fifth postulate of Euclid plays a significant role in
II. Ibn Sina's book "The Book of Healing" is one of the geometry. After Euclid Notable scientists of the world have
valuable works in his life. This work is similar to the book
been conducting research on the way to prove this postulate
"The Book of Knowledge" and is divided into large books
for several centuries. As a result of this, by the arrival of the
called "Logic", "Physics", "Mathematics" and "Metaphysics". 19th century, non-Euclidean geometry named after
The book "Mathematics" of his works consists of the sections
Lobachevsky appeared.
"Abbreviated Euclid" or the foundations of geometry,
"Abbreviated Almagest" and "Arithmetic". The "Abridged
The "Arithmetic" part of the "Book of Healing" provides
Euclid" section of this book is divided into 15 sections, and information on the methods of operations with numbers.
the information on the basics of geometry is presented in a
Here are the rules for checking the validity of methods for
similarity to the book "Inception" of Euclid. In this part, the
raising numbers using the number 9 to the values of a square
concepts of geometric figures in plane and space are given and a cube. After that, Ibn Sino designated the Indian method
and their properties are considered. It is also worth noting test using the number 9 squares with the following rules:
here that these geometrical materials are not a direct
translation of the book "Beginning", because the required If a digit is divided by 9, and the remainder is 1 or 8,
materials of mathematics in kration, expressed by their then the square of this number is divided by 9, the
opinion, enriched this part with accurate information. For remainder is 1.
example, besides the 5 postulates, Euclid gives the following
postulates: If a digit is divisible by 9 and the remainder is 2 or 7,
then the square of that number is divided by 9 and the
VI. Two straight lines contain no space. remainder is always 4.

VII. A straight line does not coincide in its direction with two If a digit is divided by 9, and the remainder is 4 or 5,
straight lines at the same time. then the square of this number is divided by 9, the
remainder is 7.
Here you can see that the V postulate and the 9-axiom of
Euclid are the same. At the same time, in this book, as well as If a digit is divided by 9, and the remainder is 3 or 6,
in "The Book of Knowledge", scientific researchers were then the square of this number is divided by 9, and the
done to prove the V postulate of Euclid in the form of a remainder is 9.
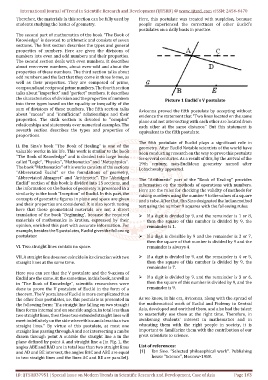
theorem. The V postulate of Euclid is more complicated than
the other four postulates, i.e. this postulate is presented in As we know, in his era, Avicenna, along with the spread of
the following form: "If a straight line falling on two straight the mathematical work of Euclid and Ptolemy to Central
lines forms internal and on one side angles, in total less than Asia, developed and enriched them, and also had the talent
two straight lines, then these two extended straight lines will to masterfully use them at the right time. Therefore, in
meet indefinitely on the side where this sum is less than two awakening students' interest in mathematics and in
straight lines." By virtue of this postulate, at most one educating them with the right people in society, it is
straight line passing through A and not intersecting a can be important to familiarize them with the contribution of our
drawn through point A outside the straight line a in the great scientists to science.
plane defined by point A and straight line a (in Fig. 1, the
angles ABE and BAD are in total less than two straight lines List of references:
and AD and BE intersect, the angles BAC and ABE are equal [1] Ibn Sino. “Selected philosophical work”. Publishing
to two straight lines and the lines AC and BE are parallel). house "Science", Moscow-1980.

ID: IJTSRD37951 | Special Issue on Modern Trends in Scientific Research and Development, Case of Asia Page 103